Tesselation Art – The Role of Mathematics in Creativity
The role of mathematics in art history cannot be overlooked, since many important mathematical elements have inspired artists for centuries to develop unique practices and visual languages that have changed the realm of Contemporary and Modern art. In this article, we will explore the multi-dimensional world of tessellation art that covers a tessellation definition, its emergence in art, its role in mathematics, and the different ways that artists have included the art form in their artwork. Read on for more about this intricate and fascinating art form!
An Introduction to Tessellation
What is tessellation? And how did it enter the art space? The term “tessellation” is commonly used in a mathematical manner, which refers to a form of tiling whereby geometric shapes are grouped repetitively without any gaps or overlapping areas. Tessellation is a process of tiling that results in a mosaic pattern or other tessellation patterns that create a mesmerizing visual effect.

A tessellation definition can also include the use of regular or semi-regular geometric shapes or tiles that are arranged to create a larger construction. The term “tessellation” is also derived from the Latin word tessellātus, which translates to “four”. The term originally referred to tessellation that was produced using square stones or pieces of clay, glass, and stone to create a basic pattern.
The Origins of Tessellation in Art
Tessellation art is similar to mosaic art in that pieces of tiles or stone are arranged to create larger compositions and are also understood as part of the decorative arts. Tessellations or tiling were first used as part of wall and architectural décor dating as far back as 4,000 BCE. The Sumerian culture was first recorded as using clay for tessellation décor in homes and venerative buildings. Other civilizations also adopted the art form to their specific cultural and artistic preferences, which helped the art form evolve.
The role of tessellation in mathematics became a primary study point for many intellectuals in the Middle Ages and the Renaissance where the use of geometric structures in nature inspired many interesting studies.
The role of tessellation in mathematics was strongly applied to architecture and existed in the form of tessellation theory. Tessellation in a mathematical context refers to tiling and the shape used repetitively to create a tessellation is referred to as a fundamental region, also known as a tile. The term “edge” refers to the intersection between two neighboring tiles, which forms a straight line while “vertex” is used to refer to the intersection of three or more neighboring tiles. These are just a few basic terms to make note of when referring to tessellation patterns.
Understanding Tessellation Patterns
The anatomy of tessellation patterns makes use of specific terminology that is useful when analyzing and interpreting the foundational elements of tessellation art. A prototile is a term used to describe the shape of the tile in a tessellation artwork. Tessellations that have only one shape of tile are recognized as monohedral tiling and can be understood in comparison to traditional paintings executed in a monochrome style (one dominant shade). Monohedral tiling or monohedral tessellations can create unique patterns when arranged in different ways. One such interesting tessellation pattern is the spiral monohedral tessellation pattern that was first discovered in 1936 by Heinz Voderberg.

Another type of tessellation arrangement is the dihedral tessellation where the tiles are arranged in a congruent manner to each other or other prototiles. This means that the tessellation pattern contains two different shapes of tiles. Additionally, with the insertion of more shapes into the tessellation artwork, the pattern and composition of the tessellation will change. Other types of tessellation patterns dependent on the number of different shapes of tiling include trihedral tessellation, tetrahedral tessellation, and n-hedral tessellation prototiles.
Tessellations are also classified according to the type of shape, which can range from regular, semi-regular, irregular, and non-regular shapes. A regular-shaped tessellation artwork would be symmetrical and composed of regular polygons. A polygon describes a figure with a minimum of three straight angles and sides with up to five or more angles. There are three types of regular tessellations, which include a network of equilateral hexagons, triangles, and squares. In a regular tessellation, the sum of the angles on a polygon would form approximately 360 degrees around each vortex.
A non-regular tessellation involves the free use of different shapes of tiles arranged in a non-regular fashion around their vertices.
Semi-regular tessellation works are composed of two or more different types of regular polygons arranged such that all vertex points are identical. This means that all vertices are surrounded by the same polygon shapes and organized in the same cyclic order. Different combinations are a product of semi-regular tessellations since eight semi-regular tessellations can be arranged in multiple combinations of equilateral hexagons, triangles, octagons, squares, and dodecagons.
Tessellation Symmetry
You may notice that certain tessellation artworks possess a level of symmetry, demonstrating the balance of proportion between two halves of each axis or object. Symmetry can also be understood as the mirror image or the imaginary line of symmetry diving into two equal parts of an object. Tessellations are also composed of different types of symmetry found in a tessellation plane. These are known as geometric transformations or plane symmetries and are divided into three different types: translational symmetry, rotational symmetry, and glide reflection.

Tessellation patterns that contain translational symmetries involve moving a figure at a set distance in a specific direction. The patterns can also be rotated around a fixed point at an angle to create rotational symmetry. The reflection of a design across a mirror line with its translation is known as a glide reflection and is the only type of tessellation symmetry that requires two steps. Together, the three types of tessellation symmetry are known as isometric and describe the tiling that does not change in size.
Wallpaper Groups
In tessellation art, patterns can be tiled to a flat surface in approximately 17 different ways. Wallpaper groups are a specific classification in mathematics founded on the symmetries of a two-dimensional design. The 17 possible tessellation patterns were tested and coined by Evgraf Fedorov in the late 19th century and later in 1924 through independent research by George Pólya. It is believed that these 17 groups of tessellation patterns were recreated in the Alhambra palace and inspired artists such as Maurits Cornelis Escher who also created a series of drawings on them.
Below, are two further categories that are used to classify tessellations according to how the unit cells with one or more tiles are organized.
Periodic Tessellations
Tessellations become periodic when the unit cells are organized in a way that produces a regular repeating pattern. Periodic tessellations often contain motifs or tiles that are repeated indefinitely in two separate directions and form motifs with symmetry produced from one of the 17 alternate wallpaper groups.
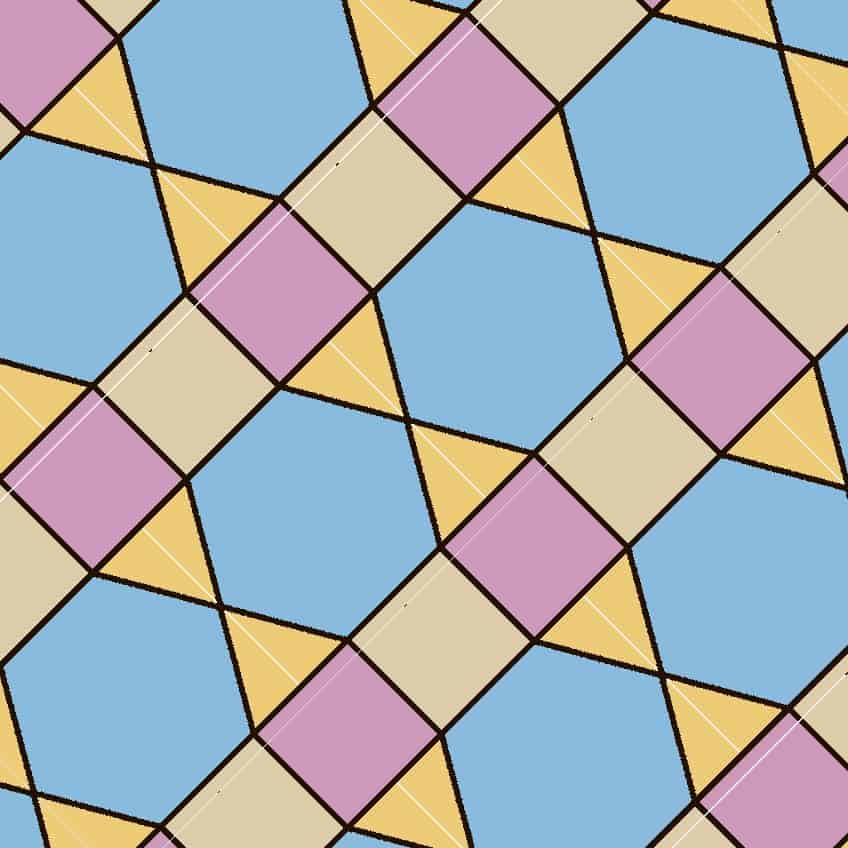
Aperiodic Tessellations
Aperiodic tessellations are arrangements that result in a random pattern or irregular pattern. Tessellations that are aperiodic contain no translation symmetry with a pattern that cannot be repeated as seen in periodic tessellations.
Examples of aperiodic tessellations include Pinwheel tilings and Penrose tilings.
Famous Examples of Tessellation Art
Now that you have an overview of the terms and classifications by which one can understand the nature of tessellation patterns, you can now enjoy some famous examples of tessellation art that demonstrate the various visuals that tessellation can produce.

Frau Nolda (1901) by Koloman Moser
| Artist Name | Koloman Moser (1868 – 1918) |
| Date | 1901 |
| Medium | Color lithograph |
| Dimensions (cm) | 24.8 x 29.4 |
| Where It Is Housed | Victoria and Albert Museum, London, United Kingdom |
Before Escher’s tessellations and creative concepts surrounding the mathematical art form, Koloman Moser was one of the pioneering artists of tessellation design in art. Many of the artist’s designs incorporate intricate motifs and patterns into wallpaper designs, posters, and even fashion and textile artworks.
Moser was a famous Austrian symbolist as well as a key founder of the Vienna Secession movement.
Moser’s works were inspired by the Art Nouveau movement, which can be seen in works such as Frau Nolda where Moser created a series of tessellation designs for woven textile art. Frau Nolda was published in a 1901 publication called Die Quelle. The color lithograph design features an organic-inspired motif repeated in alternating colors of green, brown, black, and white. At the time, Moser was also seen as the leading designer for the famous art journal Ver Sacrum. Another popular design by Moser can be found at the Steinhof Church, which was also selected as the main design for the Austrian €100 Steinhof Church coin minted in 2005.

Sky and Water I (1938) by M. C. Escher
| Artist Name | Maurits Cornelis Escher (1898 – 1972) |
| Date | 1938 |
| Medium | Woodcut print |
| Dimensions (cm) | 43.5 x 43.9 |
| Where It Is Housed | National Gallery of Art, Washington D.C., United States |
Sky and Water I is a famous tessellation artwork by one of the best-known tessellation artists of the 20th century, Maurits Cornelis Escher, whose expertise in printmaking was the perfect medium for expressing the complexities of tessellation art and the significance of such patterns. Escher’s work was inspired by his interest in tiling while employing one or more shapes. Escher’s fascination with tessellation patterns and theories was also an example of his obsession for harmony and perfection made possible through mathematics and resulted in many “trippy” tessellation artworks. Often categorized under Op art, Escher himself did not believe that his art could be classified as any movement, perhaps given the nature of his interest in harmony and balance.
His passion for tessellation patterns was more of a study and admiration for the wonders of tiling.
Escher also traveled to the Alhambra palace where he made studies of the tessellation art found on the tiling of the façade of the building. Sky and Water I is one such tessellation artwork where Escher rendered the patterns into birds and fish in an elaborate design that not only demonstrated his love for nature but also showcased his understanding of tessellation patterns through recognizable motifs.
Escher also organized his geometric shapes on a flat plane in a way that allowed the shapes to still be recognizable with appropriate spacing, especially in his black and white prints. Here he also presents an image where the viewer can choose which set of shapes to observe first, thus eliminating a clear distinction between the foreground and the background. One would have to focus on two areas of the artwork to see different shapes on their own. Escher’s art was therefore not only beautiful in a sophisticated way, but also conceptually.
Unholy 342 And Now You Know What I Know (2023) by Matt Shlian
| Artist Name | Matt Shlian (n.d. – Present) |
| Date | 2023 |
| Medium | Gold paper |
| Dimensions (cm) | 121.9 x 243.8 x 5 |
| Where It Is Housed | Artist’s collection |
Contemporary paper engineer Matt Shlian is one of the most famous tessellation artists of the 21st century who applies his expertise in commercial design, print media, and the book arts to produce magnificent and intricate paper sculptures that leave one wondering just how the artist achieves these three-dimensional tessellation artworks using paper.
Shlian repeats a single fold and describes his process as transforming from initial two-dimensional designs and drawings to three-dimensional manifestations.
Shlian has also collaborated with science experts from the University of Michigan on kinetic sculptures where he further investigates the concept of cellular division as well as solar cell development. Unholy 342 And Now You Know What I Know is one such example of a tessellation sculpture that demonstrates just how tessellation can extend beyond the two-dimensional plane and is a remarkable demonstration of the potential for further study.
Polyhex Fractal Tiling 1 by Robert Fathauer
| Artist Name | Robert Fathauer (1960 – Present) |
| Date | Unavailable |
| Medium | Digital design |
| Dimensions (cm) | Unavailable |
| Where It Is Housed | Artist’s collection |
There are many ways in which artists have creatively used tessellation ideas and theories to generate complex and stunning images and sculptures. One such artist who applied imaginative tessellation ideas to his art is Dr. Robert Fathauer. Polyhex Fractal Tiling 1 was created by visual artist Robert Fathauer whose tessellation designs have produced various fascinating visual arrangements in psychedelic colors since the 1980s.
Fathauer obtained a Bachelor of Science degree and double majored in Mathematics and Physics at the University of Denver.
Additionally, Fathauer attended Cornell University where he obtained his Ph.D. in Electrical Engineering and went on to join the research facility at the Jet Propulsion Laboratory based in Pasadena. Polyhex Fractal Tiling 1 was inspired by a fractal tiling founded by Fathauer and was the result of the arrangement of successively smaller reproductions of one prototile. The tiles in the design maintain the same shape yet differ in size. The prototile was formed by dividing a polyhex in half. A polyhex refers to a shape composed of regular hexagons connected in an edge-to-edge manner.
First inspired by Escher’s tessellations, Fathauer’s tessellation artworks began exploring fractal imagery by the 1990s and soon the artist started working digitally on his computer to realize the complex details of fractal tessellation designs. Other elements that emerged from Fathauer’s work include fractal knot designs as well as abstract sculptural forms inspired by natural designs, fractal tessellation, and hyperbolic geometry. Other inspiring tessellating artists to explore include works by Hans Hinterreiter, Woo Jeong Su, Samiur Rahman, Ekrem Yalçındağ, and Christodoulos Panayiotou.
Mathematics can be seen as the substance that helps bind the fields of art and design, which puts into perspective the many possibilities that are available for exploring new visual narratives and techniques. Tessellating artists, such as M. C. Escher and Koloman Moser, were foundational to the discovery of the potential visual narratives that could be harnessed from tessellation theories. Other popular examples of tessellation art can be found at the 14th-century Alhambra palace in Granada, Spain, the Arab Hall at the Leighton House Museum in London, and the Moroccan Court set up at the Metropolitan Museum of Art in New York City.
Take a look at our tessellation in art webstory here!
Frequently Asked Questions
What Is Tessellation Art?
Tessellation art can be defined as artwork that mimics tessellation theories and geometrical tiling arrangements as found in mathematics to create designs using regular, semi-regular, irregular, and non-regular geometric shapes. These shapes can be arranged in ways with no gaps between their opposing sides. Another term for tessellation is tiling.
What Makes Tessellation Art Significant?
Tessellation art is regarded as significant due to its nature of combining concepts from mathematics and art to create visual arrangements and designs that can only be created with specific techniques. Tessellations are also versatile as they can be applied across multiple fields, including architecture, interior design, textiles, and decorative arts, while holding mathematical value.
When Did Tessellation Art Begin?
Tessellation art began around 4,000 BCE with decorative clay tiling designs found in Sumeria. Tessellation was also applied in mosaic art in ancient Rome as small clay, stone, or glass pieces of tiling to form a larger image.
Jordan Anthony is a Cape Town-based film photographer, curator, and arts writer. She holds a Bachelor of Art in Fine Arts from the University of the Witwatersrand, Johannesburg, where she explored themes like healing, identity, dreams, and intuitive creation in her Contemporary art practice. Jordan has collaborated with various local art institutions, including the KZNSA Gallery in Durban, the Turbine Art Fair, and the Wits Art Museum. Her photography focuses on abstract color manipulations, portraiture, candid shots, and urban landscapes. She’s intrigued by philosophy, memory, and esotericism, drawing inspiration from Surrealism, Fluxus, and ancient civilizations, as well as childhood influences and found objects. Jordan is working for artfilemagazine since 2022 and writes blog posts about art history and photography.
Learn more about Jordan Anthony and about us.
Cite this Article
Jordan, Anthony, “Tesselation Art – The Role of Mathematics in Creativity.” artfilemagazine – Your Online Art Source. October 17, 2023. URL: https://artfilemagazine.com/tesselation-art/
Anthony, J. (2023, 17 October). Tesselation Art – The Role of Mathematics in Creativity. artfilemagazine – Your Online Art Source. https://artfilemagazine.com/tesselation-art/
Anthony, Jordan. “Tesselation Art – The Role of Mathematics in Creativity.” artfilemagazine – Your Online Art Source, October 17, 2023. https://artfilemagazine.com/tesselation-art/.